A tool to explore the energy landscape of magnetic materials
By Nicola Nosengo - NCCR MARVEL
Codes based on Density Functional Theory (DFT) and its modifications are staple tools for computational materials scientists, because they allow to explain and predict many fundamental properties of materials by calculating how the electrons of the material are arranged across orbitals and energy levels.
DFT works by looking for the electronic structure with the lowest energy, the material’s ground state. But this search can be particularly complicated for magnetic materials, which often have many possible solutions to the electronic structure problem. In addition to the actual ground state there can be local minima, or metastable solutions, that are states where the system can end up and stay in particular conditions, even if they do not represent the lowest possible energy state.
Finding all the possible local minima, as well as the true ground state, depends on being able to start a DFT calculation from many different points of the departure. “But the way DFT typically works is that the code chooses a starting occupation of the orbitals, and then you let it go and optimize the energy, but you have no real way to tell the code that you want to start from a different point”, says Louis Ponet, a scientist in Nicola Marzari’s MARVEL laboratory at EPFL. “You are at the mercy of how the code was written”.
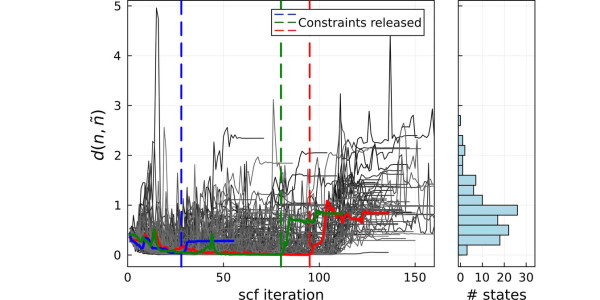
Distance to target occupation matrix during self-consistent cycle. From Ponet et al., npj Comput Mater (2024).
To solve the problem Ponet, Enrico Di Lucente and Nicola Marzari have developed a new computational method that allows to perform this kind of thorough exploration of the energy landscape of magnetic materials, accessing many different starting points in terms of which orbitals are occupied by the electrons, and then look for the local minima much more granularly that how it’s currently done. The method, as well as its validation on many magnetic systems, has just been published in npj computational materials.
One key element of the method was the addition of mathematical tools called Lagrangian multipliers that, when appropriately fine-tuned, allow to drive the system to the desired occupation of orbitals and subsequently accompany it to the closest self-consistent minimum. “Mathematically, the multipliers are a way to shift your parabola on one side or the other to move closer to a local minimum” says Ponet. “But you have to do it right and optimize your multiplier together with all the other parameters in your state. You need a code that updates the multipliers simultaneously with the charges and other DFT parameters and does that with the same method”. The other important part of the study is a global search algorithm that allows to search systematically for all possible local minima of the energy in a material.
“This method spans the whole range of possible occupations of orbitals, where you can have all possible spin configurations” says Enrico Di Lucente, a PhD student in Marzari’s lab and co-author of the paper. “Acting on the orbitals lets you see not just what the magnetic moment is, but how the electrons are building it”.
The group first tested their method on nickel oxide – NiO, and its supercell variants N2O2 and Ni4O4. “It’s a very simple material in terms of local occupation, and its degree of freedom for filling the orbitals is not high” says Ponet. Then they moved on to manganese oxide, that has more freedom and more complication, as well as other oxides. “We also studied magnetism from p-orbitals in the SrN system and found that the global search is essential for obtaining the ground state of the rocksalt phase” says Di Lucente. “As well as cases where mixed-valence orbital occupations could be present, like in the case of skutterudites”. They applied the method in combination with different widely used DFT functionals, including PBEsol, PBEsol + U, PBE0 and HSE.
Overall, the method proved capable of revealing a complex landscape of energy states, or a “zoo of metastable electronic states”, as the authors write, for each of these materials. “When you are done with the search you are 99,9% sure that you’ve found the real ground state, as well as the first excited states that are immediately above” says Di Lucente. “This is very useful if you are working with experimentalists, and they find a new state that you want to map on the material’s energy landscape”.
And the applications of the method are not limited to magnetic materials, notes Ponet. “The method per se can be used also to force materials into some charged states, moving charges around orbitals and not necessarily orbitals with different spins” he says. “It provides knobs to force charges into specific places or electrons to occupy specific orbitals, to force a material in a certain state and see if it stays there”.
The group will now keep working to expand the high-throughput capability of the method. One key point will be to come up with smart ways to create new useful starting points for the algorithm, and the group is now exploring the possibility of using machine learning to suggest what states to try next while exploring the energy landscape of a given material. “It could be a big improvement, but at the moment it works well for some materials and not so much for others”, says Ponet.
Reference
Ponet, L., Di Lucente, E. & Marzari, N. The energy landscape of magnetic materials. npj Comput Mater 10, 151 (2024). https://doi.org/10.1038/s41524-024-01310-w
Low-volume newsletters, targeted to the scientific and industrial communities.
Subscribe to our newsletter